As θ → 0, we have cosθ → 1
Proof :
When θ = 0, We have, lim\(_{θ\to 0} \cos \)θ = cos0 = 1 { ∵ cos0 = 1 }
Hence,
ADVERTISEMENT

As θ → 0, we have cosθ → 1
Proof :
When θ = 0, We have, lim\(_{θ\to 0} \cos \)θ = cos0 = 1 { ∵ cos0 = 1 }
Hence,
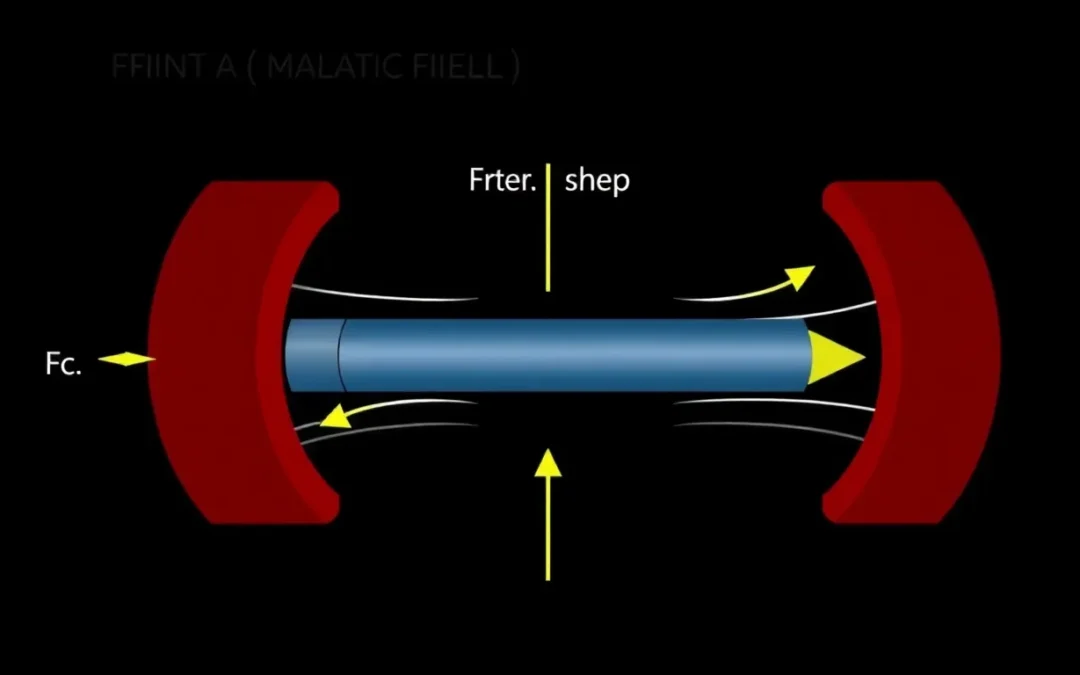
Sharpen magnetism applications.
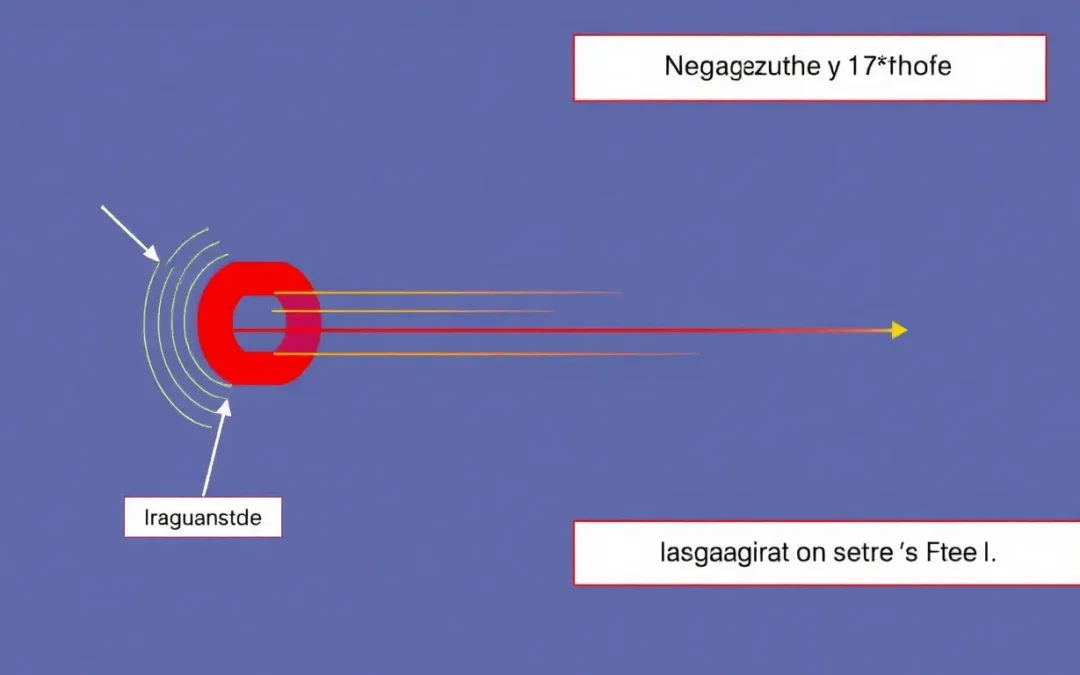
Excel in magnetic field numericals.
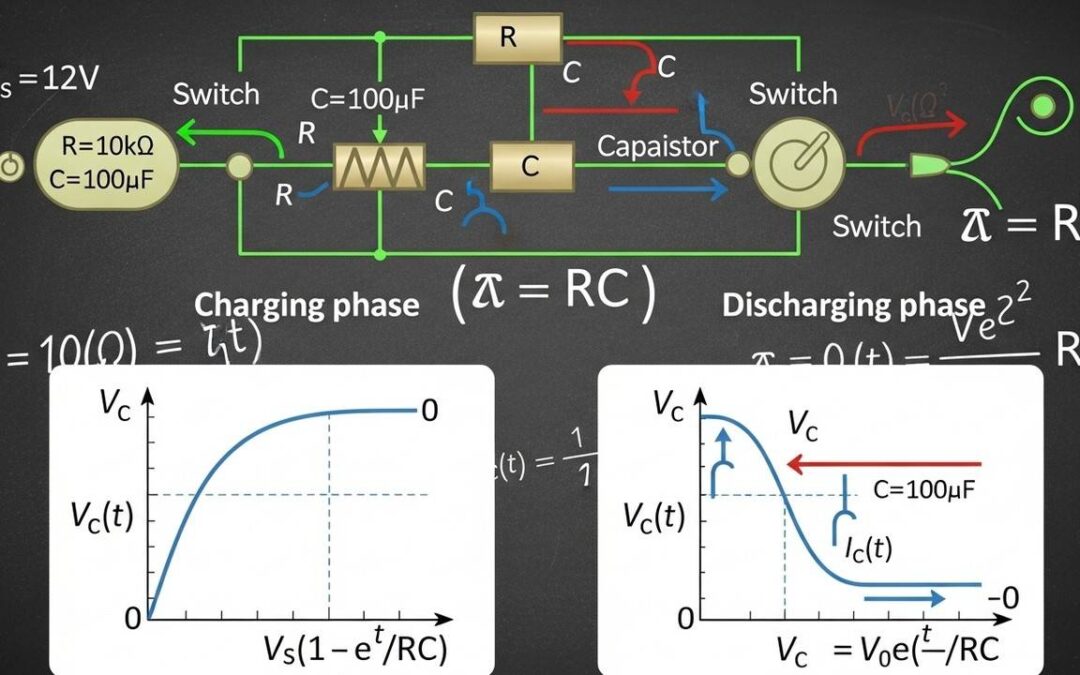
Master transient analysis in RC circuits.
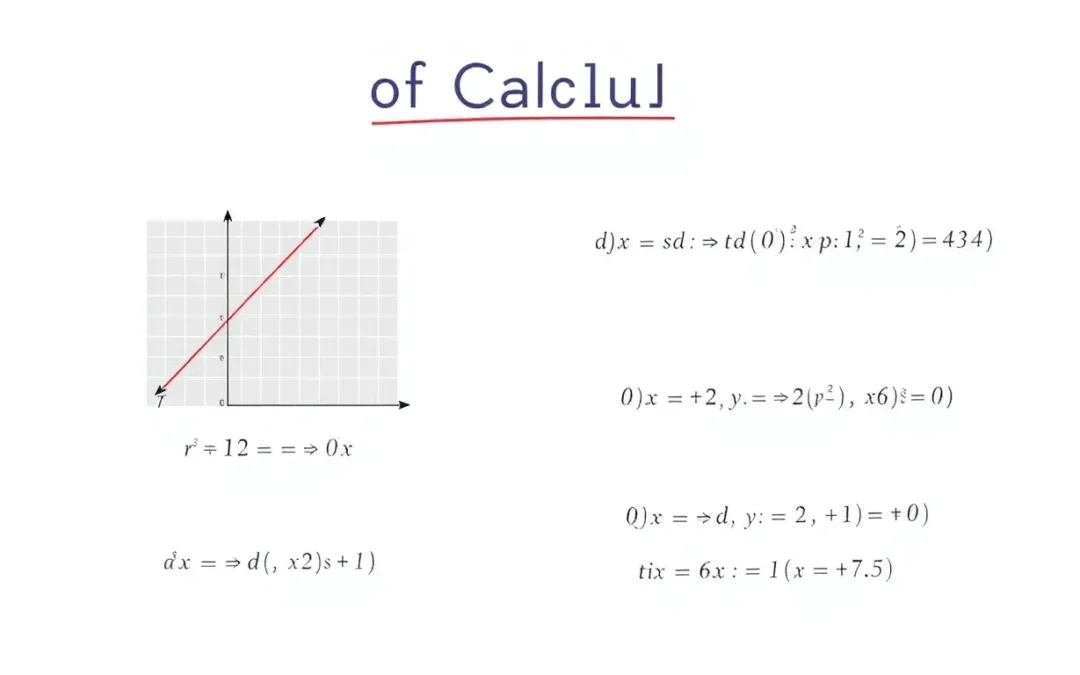
Learn how to solve Evaluating Limits Problems with this step-by-step guide. We’ll cover the fundamentals and provide clear examples.

Learn how to solve Limits at Infinity with this comprehensive guide. Understand the concepts and techniques through clear examples and step-by-step solutions.
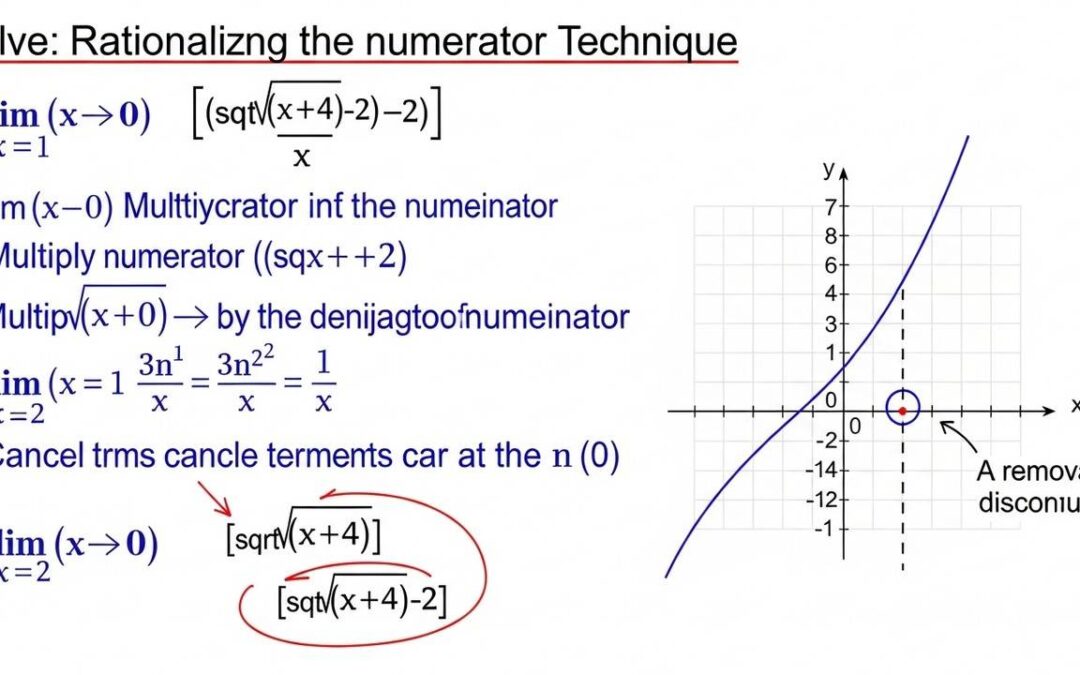
Learn how to solve Rationalizing Numerator Limits by rationalizing the numerator to eliminate indeterminate forms and find the limit.
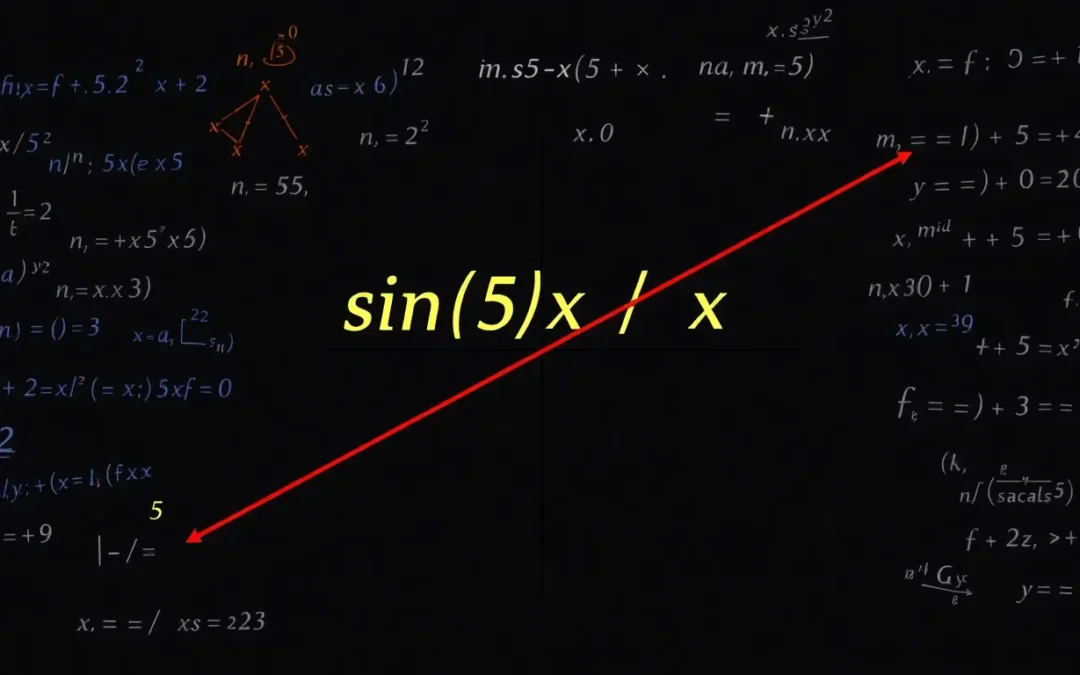
Learn to evaluate the Trigonometric Limit. The solution involves simplifying the expression and applying limit theorems. The final result is 5.
0 Comments